HomeAlgebraFunctionHow to Find the Domain of a Function
September 1, 2013

How to Find the Domain of a Function

The domain of a function in x refers to all the possible values of x for which the function is defined. That is, it consist of all the values that make the function exist. Unless otherwise stated, the possible values for the domain is from the set of real numbers. Usually, your teacher will only ask you to find the domain of a rational function. For example, if you have a function defined as f(x) = \frac{4}{2x} then its domain is the set of all real numbers except 0. You can write this as D: {x/x?0}. This is read as “The domain is the set of all x such that x is not equal to zero.  Why is zero not included in the domain of f(x)? This is because if x = 0, then you will have f(0) = \frac{4}{0}. Division by zero is not defined. The function does not exist at x = 0 for this function.

Practice

Domain of a function

 

 

 

 

 

 

 

 Solution

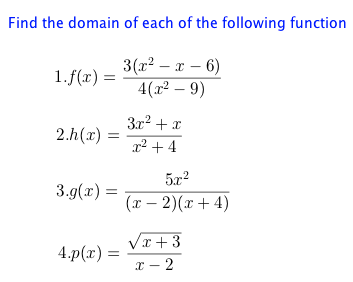
1. g(x) = \frac{5x^2}{(x-2)(x+4)}

Answer: D: {x/x?2, x?-4}. This means that the function is defined in the set of real numbers except when x = 2 or when x = -4. At these values, the denominator will be 0.

2. h(x) = \frac{3x^2+x}{x^2+4}

Answer: D: {x/x ? R}. This means the domain is the set of real numbers. There is not number that will make the denominator equal to 0.

3. f(x)= \frac{3(x^2-x-6)}{4(x^2-9)}

Wrong answer: \frac{3(x-3)(x+2)}{4(x-3)(x+3)}=\frac{3(x-2)}{4(x+3)}. Therefore D:{x/x ? -3}.

Correct Answer: If 4(x^2-9) = 4(x+3)(x-3)=0, then x = -3 or x = 3. The function will not exist at this values of x because it will make the denominator 0. So the domain is D:{x/x ? -3, x ? 3}.

4. p(x)= \frac{\sqrt{x+3}}{x-2}

Answer: D={x/x ? 2, x ? -3}.

Where did the x ? -3 come from? This came from the numerator. The values of x cannot be less than 3 because it would mean that we will have a square root of a negative number. The square root of a negative number is not defined in the set of real numbers.

Finding the domain is a little bit tricky. This is because when you are asked to find the domain of a function, you want to know all the possible values of x that make that function exist. Yet, what you write in the domain, after saying all x, you oftentimes write the restrictions for the values of x that make the function not exist! So, be careful.

Three things to remember in determining the domain of a function
  1. The domain is the set of all possible value of x that make the function exist.
  2. The values of x that makes the denominator equal to 0 is not included in the domain.
  3. The values of x that makes the value under the square root sign negative is not included in the domain.
Tags:,

Related Posts

About The Author

lines